| 第22行: | 第22行: | ||
<q> | <q> | ||
Specifies transitivity on <c. Let | Specifies transitivity on <math><_{c}</math>. Let <math> o_{1} <_{c} o_{2} </math>. Then | ||
following rule ensures, that there is no o that is greater | following rule ensures, that there is no <math>o</math> that is greater | ||
than | than <math>o_{2}</math>, but smaller than <math>o_{1}</math>, with respect to <math><_{c}</math> | ||
</q> | </q> | ||
2024年8月13日 (二) 07:38的版本
Notes on YATA paper
This is notes on Near Real-Time Peer-to-Peer Shared Editing on Extensible Data Types[1].
Rules
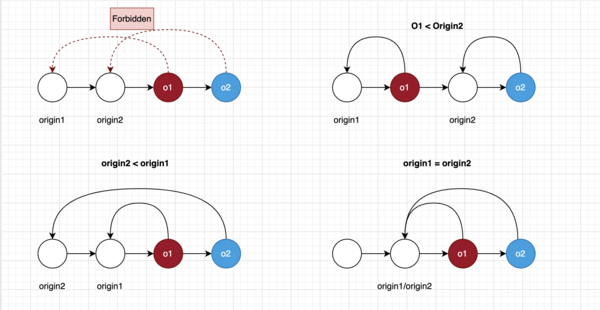
Rule1: No line crossing
Rule1: We forbid crossing of origin connections (red lines
in the graphical representation) between conflicting insertions. This rule is easily explained using the graphical representation of insertions in the linked list. As we stated
before, every insertion has an origin connection to an insertion to the left (to a predecessor). Only when two operations are concurrently inserted after the same insertion,
they will have the same origin.
[math]\displaystyle{ o1 \lt _{rule1} o2 ⇔ o1 \lt origin2 ∨ origin2 ≤ origin1 }[/math]
- Red line refers to the connection from the operation to it's origin
- The assumation is that o1 and o2 conflicts, which means that there's overlap between intentions of them.
Rule2: transitivity on <c
Specifies transitivity on [math]\displaystyle{ \lt _{c} }[/math]. Let [math]\displaystyle{ o_{1} \lt _{c} o_{2} }[/math]. Then
following rule ensures, that there is no [math]\displaystyle{ o }[/math] that is greater
than [math]\displaystyle{ o_{2} }[/math], but smaller than [math]\displaystyle{ o_{1} }[/math], with respect to [math]\displaystyle{ \lt _{c} }[/math]
[math]\displaystyle{ o1 \lt _{rule2} o2 \iff \exists o : o2 \lt c o \to o1 ≤ o \iff \nexists o : o2 \lt _{c} o \lt o1 }[/math]